This unit calls upon understandings from all three grade levels in middle school CCSS. Later lessons, in particular, ask students to apply skills and understandings from grade 8 CCSS. Since this unit falls at the beginning of the 8th grade year of the OpenSciEd Scope and Sequence, it’s important to identify which math concepts and skills students may need extra support in applying as these concepts are fundamental to students engaging in Science and Engineering Practices like analyzing and interpreting data as well as using mathematical and computational thinking.
Mathematical concepts and skills from middle school CCSS are used in the following lessons:
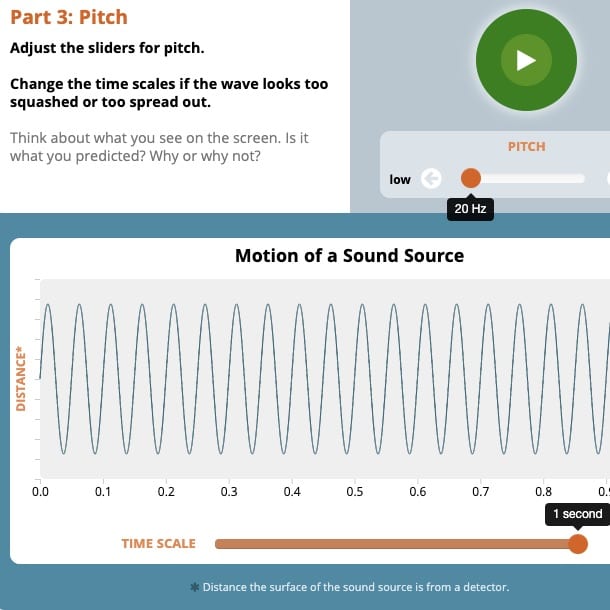
- In Lesson 4, students collect and analyze data in the form of distance vs. time graphs showing the motion of a vibrating stick over time. Students characterize the shape of these graphs as wave patterns, and describe differences in properties like the vertical distance between peaks and troughs of waves when looking at graphs for louder vs. softer sounds. They will also connect the properties of these functions to the physical differences they represent using the axes of the graph to inform what the graph represents. This data analysis calls on the ability to describe the relationship between two quantities using a graph (CCSS.MATH.8.F.B.5) as well as the ability to compare two functions expressed graphically in order to determine key properties about each function (CCSS.MATH.8.F.A.2).
- In Lesson 5, students are expected to exercise these same skills and understandings to interpret differences between graphs of distance vs. time for a stick simulating higher- and lower-pitched sounds. In addition, students define the frequency as the amount of vibrations the stick goes through in a second. They calculate this frequency by finding the unit rate (vibrations per second) using the overall number of vibrations and the total time passed (CCSS.MATH.6.RP.A.2).
- In Lesson 5 and 6, students work independently to interpret graphs of time vs. distance to describe sounds in terms of pitch and loudness using qualitative properties of the functions shown on each graph (CCSS.MATH.8.F.A.2). Depending on the math understandings that students display in Lessons 4 and 5, students might need support in reading and interpreting these graphs. It may help some students to go over the axes of the graph as a class to draw attention to what the graph is showing, and students may benefit from probing questions like, “What differences do you notice between these graphs? How do those differences compare to what we saw in the graphs we made with the motion detector?”.
- In Lesson 13, students discuss and use mathematical methods of finding the average of data sets, including calculating mean and median as a way to combine results from different groups in order to improve the accuracy of the class’s data. They also work together as a class to decide how to account for or discard outliers in the class’s data in order to best represent what each group found in their investigations (CCSS.MATH.6.SP.B.5). Depending on their experience using these concepts in math classes, students may need reminders of how mean and median are calculated. You can support students in recalling these procedures by taking a sample data set (either from the investigation or a random example set) and working together as a class to describe how students could find the mean and median of the set. By doing this with the class or with small groups that could use this extra practice, you can support students with mathematical methods needed to analyze the data the class has collected to draw conclusions about the lesson question.
- Later in Lesson 13, students gather data describing how the energy of a vibration changes with changes to the frequency and amplitude of the vibration. They then use this data to describe and graph functions that represent the relationships between energy and frequency and energy and amplitude. From their numerical data and the graphs they create, students will see that there is a proportional relationship between frequency and energy transferred; when we increase the frequency of the vibrations, the energy transferred increases in proportion (CCSS.MATH.7.RP.A.2). While frequency and energy have a linear relationship, amplitude and energy have a nonlinear relationship where increasing amplitude causes much greater increases in energy compared to increasing frequency. Some students may recognize the pattern on the amplitude vs. energy graph as exponential, where increasing amplitude causes a much greater increase in energy than in a linear relationship like that between frequency and energy (CCSS.MATH.8.F.A.3).
- By the end of Lesson 13, Students are expected to use these qualitative properties of the graphs for frequency vs. energy and amplitude vs. energy to conclude that the energy vs. amplitude function has a greater rate of change than does the energy vs. frequency function (CCSS.MATH.8.F.A.2). Depending on students’ experience with linear and nonlinear functions, students may identify these differences in different ways. Some students may only be able to state that the amplitude vs. energy graph is steeper or increases more quickly, and they may need support in connecting this observation to the idea that increasing amplitude causes a greater increase in energy compared to frequency. Further, some students may benefit from using the data tables they generate in their investigations to find numerical patterns to support their observations of the two graphs. In this numerical data, for example, they might notice that doubling the frequency doubles the energy but doubling the amplitude makes the energy increase by 4 times. These numerical patterns may be helpful to call out and emphasize for students who are still developing their skills at reading and analyzing graphs.
